---

24 December 2018 | Draft

Engaging with Elusive Connectivity and Coherence

Global comprehension as a mistaken quest for closure

-- / --

---

Introduction
Imagining the Flag of Europe otherwise?
Borromean challenge to comprehension of any trinity?
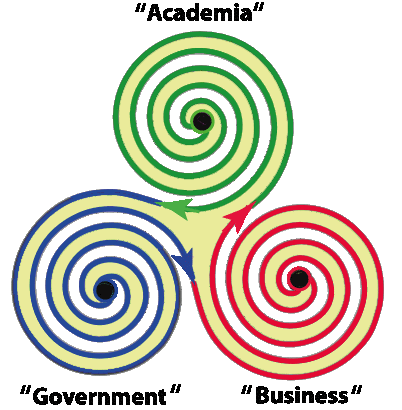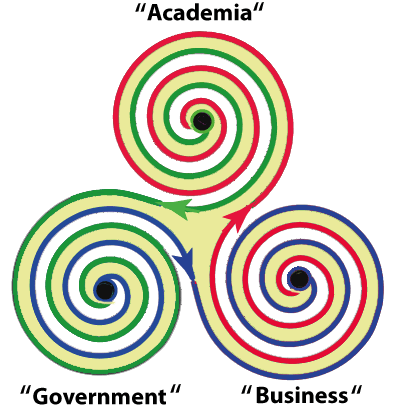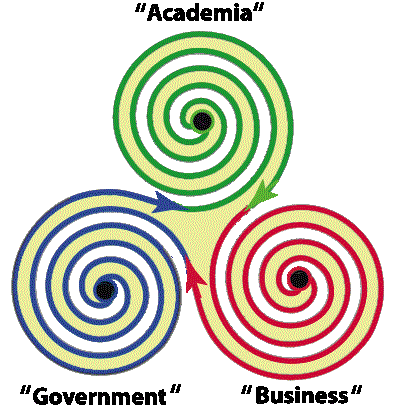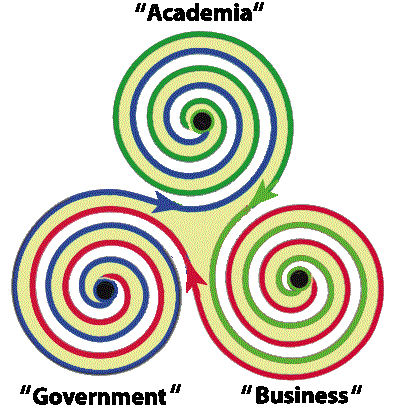
Requisite curvature: reconciling the Triple Helix, the Triskelion and the Borromean condition
Necessary cognitive twist: star symbols as bladed propellers -- for propulsion in 3D?
Direction of propulsion in a global context -- as enabled by configuration of symbolic stars
Engaging globally with knots and riddles -- Gordian and otherwise
Conversation theory, actor interaction and boundaries
Comprehension of elusive connectivity and coherence for sustainable governance
Unrecognized reminder of globality from the focus of ball games
Constraints on comprehension and communication: technology and intellectual property
Engaging experientially with potential: anticipation and nostalgia
References

---

Produced on the occasion of the unexpected uprising of the Gilets Jaunes ("Yellow Vests") throughout France,
with images of Paris in flames, symbolically paralleled by a UN Climate Change Summit to implement the Paris Agreement

---

Introduction

There is a curious confluence of disruptive dynamics in this period. These include the confusion surrounding the implementation of the UN Climate Change Agreement and that associated with the Global Compact for Safe, Orderly and Regular Migration -- opposed by populist movements associated with right-wing agendas. Symptomatic of the disruption, and a symbol of a wider challenge to democratic processes, is the uprising in France by the Gilets Jaunes ("Yellow Vests") -- recently contained there by the "forces of law and order" who are themselves threatening their own uprising at the time of writing. This dynamic can be readily seen as modelling challenges faced by the European project as a whole. It can also be seen as indicating the forthcoming response to implementation of the Climate Change Agreement (Andrés Ortega, "Yellow Vests": The First Rebellion Against the Ecological Transition, The Globalist, 4 December 2018).

A number of countries are now witness to similar internal dynamics, possibly characterized as "poisonously" divisive -- as in the USA and the UK -- curiously accompanied by political ambitions to "be great again". To such processes it is appropriate to add the escalating USA-Russia and USA-China tensions, as well as the continuing regional conflicts and proxy wars, most notably in the Middle East. Other conflicts and challenges are foreseen in relation to resources. Also foreseen is yet another financial crisis as a consequence of issues unresolved since the previous occasion.

The following argument follows from a previous exercise in endevouring to reframe the divisive governmental response to "wings" in the political system, notably those deprecated as radical and extremist (Coordination of Wing Deployment and Folding in Politics: bird flight and landing as complementary metaphors of global strategic coherence, 2018). With respect to that metaphor, fruitful reflection is challenged by excessive value attached to being at the "leading edge" -- deprecating those on the "trailing edge" -- when both are essential to flight (Seeking the "Cutting Edge" of Sustainable Community, 1997).

Significantly, the uprising of the Gilets Jaunes is giving rise to a truce period in which a Grand Débat national is being organized in France at all levels of society. It remains completely unclear whether the capacity for such dialogue can engender the coherence for which many hope. Specifically are the available skills for such dialogue adequate to the tasks or yet to be recognized as "not fit for purpose"?

That earlier document endeavoured to interrelate various threads, most notably the star symbols so systematically displayed on national flags before which leaders declaim their strategic ambitions. Such symbols lend themselves to visualization in three-dimensions as both "wings" and as "propellers". The visualizations suggested the merit of re-imaginging star-filled flags, such as the Flag of Europe, in order to determine whether they could be presented dynamically in 3D to indicate greater possibilities of collective empowerment such as to enable "lift off" and sustainable "flight" of psycho-social systems, as in the case of Europe. With respect to the global response to climate change, how is psycho-social "propulsion" to be understood otherwise -- or is it dangerously assumed that no new thinking is required on such matters?

The argument here is based on the recognition that there is a major disconnect between what is highly valued as a symbol -- whether articulated in terms of birds (eagles, etc) or stars -- and the lived experience of many "on the ground". In transcending partisan perspectives, such symbols would appear to be unrelated to the reality of partisan dynamics. There is considerable irony to the fact that many animals are valued as national symbols at a time when degradation of the environment is threatening their extinction -- aside from any consideration of the biodiversity which the relations between those symbolic animals might otherwise imply.

The approach in what follows is to see the challenge as a challenge to collective imagination which can benefit from new metaphors (In Quest of Mnemonic Catalysts for Comprehension of Complex Psychosocial Dynamics, 2007; Imagining the Real Challenge and Realizing the Imaginal Pathway of Sustainable Transformation, 2007; Global Challenge of the Global Challenge: ¿ In-quest of a decision-making framework appropriate to a world in crisis? 2016).

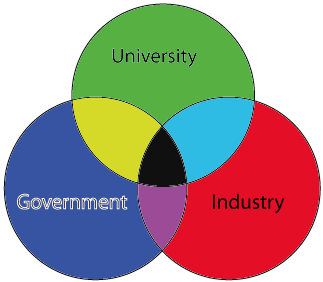
In the quest to elicit cognitive empowerment from fundamental symbols, threads which merit interweaving include: bird flight (as an inspiration for lift-off), propeller design (as a motivating force), the Triple Helix model of innovation (interrelating government, academia and business, for example), and visualization technology (offering insights into 3-dimensional dynamics). These threads were introduced with animations in the earlier document.

The challenge here is to take the argument further in the light of insights into engaging with the connectivity and coherence they so elusively imply. Specifically the concern is with how the quest for closure, as reflected in the strategic quest for sustainability, peace, and the resolution of various problems, may be mistaken as a quest for stasis consistent with the ill-adapted conventions of the "state" (nation state, state of the union, state of the world, state of the future), as previously argued (Dynamic Transformation of Static Reporting of Global Processes, 2013). The dynamics of the world would indeed appear to call for a form of process thinking.

However the additional twist to be explored is the possibility that insight is not "deliverable" statically as a product by new technology. Rather it is intimately associated with experimental and experiential engagement with such facilities. This can be seen as a feature of use of social media, exemplifying the early insight of Marshall McLuhan (The Medium is the Message, 1967).

Consistent with this perspective is the challenge of communicating coherence when the process of experiential engagement is necessary to any insight -- only briefly to be "grasped", as can be provocatively argued (Beyond Harassment of Reality and Grasping Future Possibilities: learnings from sexual harassment as a metaphor, 1996). Expressed otherwise, the challenge of comprehension and coherence might be better framed as one of learning to dance -- coming together and drifting apart

Unity, and the desperate collective quest for it, could better be understood as an illusion, with greater insight offered by liminality (The Consensus Delusion: mysterious attractor undermining global civilization as currently imagined, 2011; Living as an Imaginal Bridge between Worlds: global implications of "betwixt and between" and liminality, 2011). This frames the need to engage more fruitfully with incomprehension (Living with Incomprehension and Uncertainty: re-cognizing the varieties of non-comprehension and misunderstanding, 2012)

These issues are highlighted by the following argument and the constraining uses of current visualization technology -- anticipating the future possibilities of virtual reality.

Imagining the Flag of Europe otherwise?

The earlier argument made a case for exploring new designs for the Flag of Europe in the light of the symbolic and cognitive function of stars and their relation to flight -- as winged birds.

The case for such exercise also follows from earlier arguments (Animating the Representation of Europe: visualizing the coherence of international institutions using dynamic animal-like structures, 2004). The examples below are merely indicative of some possibilities, whether or not the emphasis is placed on a 12-star flag or a 28-star flag (possibly plus or minus 1, as a consequence of Brexit and future adhesions).

All 28 stars rotate in all examples. In that on the left the circle as a whole rotates. The stars can be enlarged so that alternative stars interlock in their rotation (in contrast with the simplest case). The ring of counter-rotating stars can be presented as 3 mutually orthogonal rings.

Design exercises for animated 28-country Flag of Europe based on 28 rotating stars
Simple rotating circle of 28	Counter-rotation of alternate stars	3-orthogonal circles of 28	Borromean ring configuration

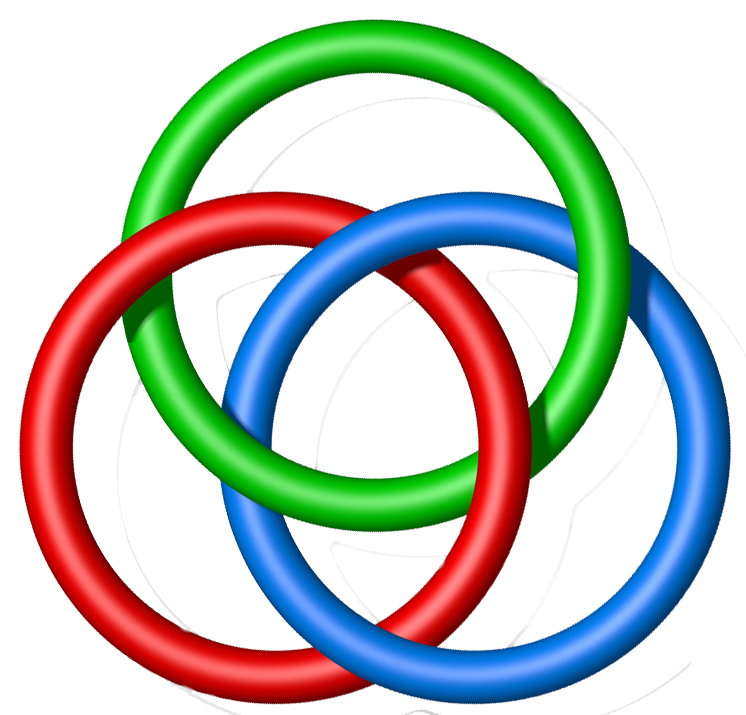
With some adjustment, the rings can be made to interlock (or interlace), as could be inferred from the animation on the right (above) -- understood as a Borromean ring configuration. This is of great significance in logic and mathematics. It has been chosen as the logo of the International Mathematical Union, as in the design by John M. Sullivan (New IMU Logo based on the tight Borromean rings, 2006; Charles Gunn and John M. Sullivan, The Borromean Rings: a video about the new IMU Logo, 2008). Their relevance to the coordination of socio-political systems is typically ignored, whatever role they may have in dialogue informed by symbolic dimensions.

Examples of 3-fold articulations of Borromean rings of relevance to coordination of political systems?
Representation of rings interlocking according to the Borromean condition
Early depiction of Christian Trinity	Common representation in 2D	Distorted representation as ellipses	3D representation	International Mathematical Union Logo
Reproduced from Wikipedia

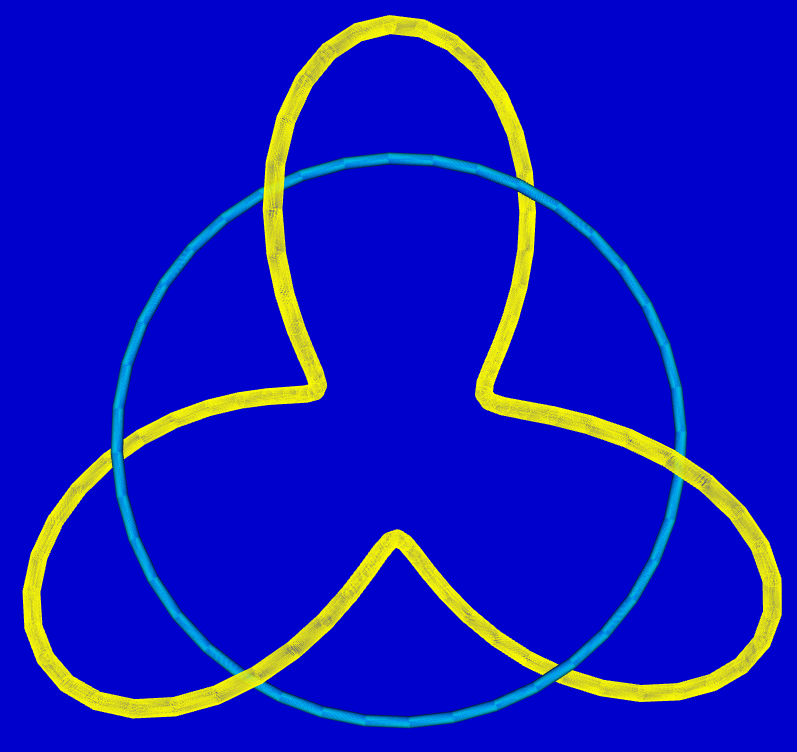
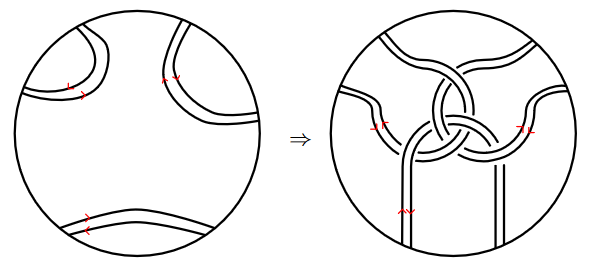
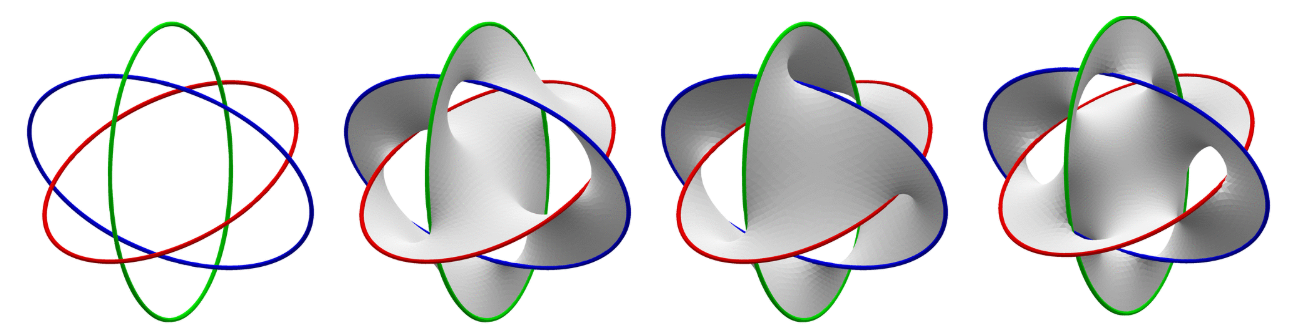
Of particular significance to this argument however, as configured in the Flag of Europe animations above, the "Borromean rings" depicted there are not correct since any two rings are interlocked with each other (corresponding to what is recognized as the (3,3)-torus link).. By contrast Borromean condition issuch that each two rings are not interlocked with each other -- but the three rings as a whole are connected and not separable. True Borromean rings are connected, but when any one is cut and removed, the remaining two rings are not connected. The three rings as a whole are connected and not separable.

The image most commonly used in 2D to illustrate the Borromean condition (second from the left, from Wikipedia) is therefore potentially completely misleading. This is conformed by the name given to the image by its designer (Jim Belk, Borromean Rings Illusion, Wikipedia). A geometrically correct 3D representation of Borromean rings is not possible using circular rings. The rings have to be either non-planar, namely rather wavy, or to be ellipses rather than circles.

Borromean challenge to comprehension of any trinity?

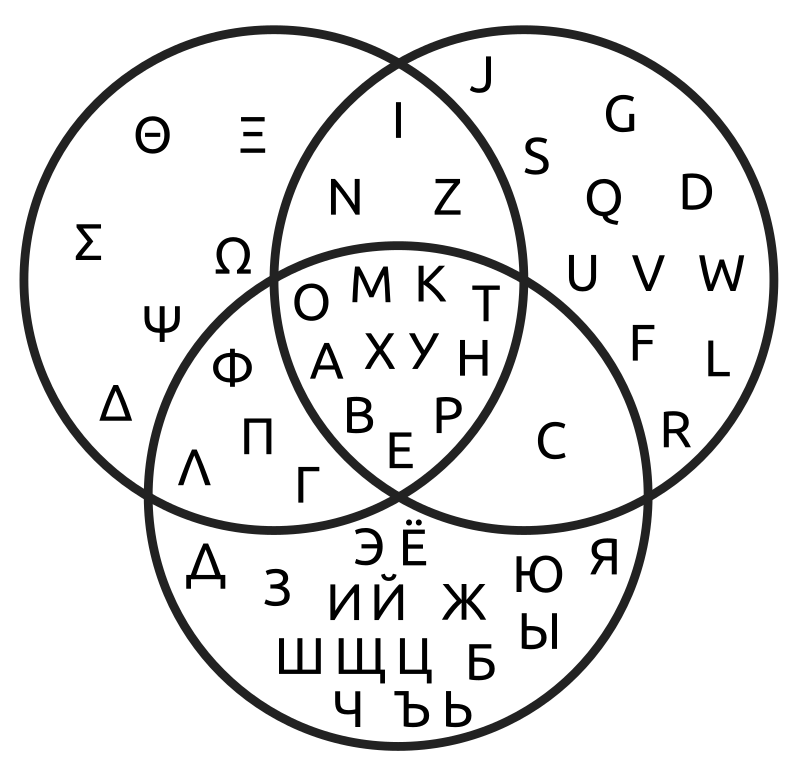
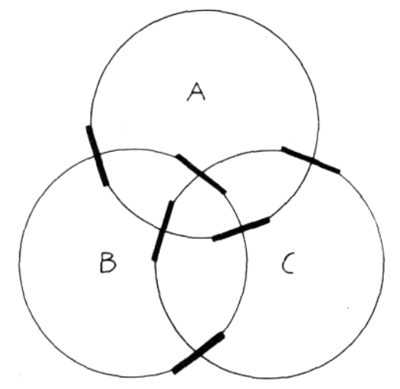
The images above serve to highlight a challenge to comprehension of any trinity. The least controversial is of course the Venn diagram, as shown below.

Venn diagram
Showing the uppercase glyphs shared by the Greek, Latin, and Cyrillic alphabets	As a truth table
Reproduced from Wikipedia

Far more controversial is the comprehension of the Christian Trinity to which mystics allude, as discussed and illustrated separately (Vlad Alexeev, Borromean Rings, Impossible World; Symbols of the Holy Trinity, Holy Trinity Amblecote; Borromean Rings, ThisIsChurch.com). The question is whether its integrative function of 3-in-1 and 1-in-3 is best to be presented in terms of a Venn diagram or a Borromean condition. The medieval depiction above in 2D is indicative of an aspect of the challenge since it can be readily misunderstood in terms of the former rather than the latter.

This distinction is most clearly made and illustrated in an extensive analysis of how Dante Alighieri describes the three rings (tre giri) of the Holy Trinity in Paradiso 33 of the Divine Comedy (Arielle Saiber and Aba Mbirika, The Three Giri of Paradiso XXXIII, Dante Studies, 131, 2013, pp. 237-272). The remarkable interdisciplinary exploration combines insights from speculative theology, geometry and knot theory. It is of particular relevance to the argument here, especially in relation to knots (as discussed further below).

(3,3)-torus link (circles do not need to bend to form the 3-link, see knot atlas)	Borromean rings (circles must bend to be able to be woven together)
Reproduced from The Three Giri of Paradiso XXXIII (2013)

Especially provocative is whether the interrelationship between the three main Abrahamic religions (Christianity, Islam, Judaism) is appropriately represented as a Venn diagram or rather by a Borromean condition, given what they purportedly hold in common -- particularly since the challenges of that relationship are inadequately "contained" by the simplicity of the former. Aspects of the arguments are presented separately (Reconciling Symbols of Islam, Judaism and Christianity: catalytic methodology for effective interfaith dialogue, 2017).

In the emerging "post-truth" era, the example above of use of a Venn diagram as a truth table usefully raises the question as to its adequacy with respect to the surreal nature of the times, as discussed separately (Surreal nature of current global governance as experienced, 2016; Towards articulation of a "post-truth table"? 2016). Is greater subtlety held by a representation of the Borromean condition -- in "non-tabular" form?

These questions help to frame the question as to what is meant by the "unity" for which national, religious and world leaders so frequently appeal. Do they understand what might be meant by that? Is it too readily assumed that everyone understands its nature? Further subtlety is offered in the cited analysis of Dante's Divine Comedy (Saiber, 2013). Aside from its relation to appeals for peace or sustainability, what meaning does unity have in relation to the ecosystem and biodiversity? Does reference to and holiness and holons avoid the question of how these are to be comprehended in practice -- however extensive the arguments of David Bohm and their application by him to dialogue (Wholeness and the Implicate Order, 1980; 1996; On Dialogue, 1996)?

Of some relevance to such questions is the notion of "wholth" (Wholth as Sustaining Dynamic of Health and Wealth: cognitive dynamics sustaining the meta-pattern that connects, 2013; Encycling, enwholing and wholth, 2014). The term is a focus of a thesis by John C Samples (Wholth : a philosophy of religious education, Emmanuel School of Religion, 1972).

Requisite curvature: reconciling the Triple Helix, the Triskelion and the Borromean condition

The potential cognitive complexity of any trinitarian condition of 3-fold coordination is usefully indicated by the Triskele (or triskelion), an ancient Celtic symbol illustrated on the left below, and discussed separately (Cognitive Implications in 3D of Triadic Symbols Valued in 2D: representations of the triskelion in virtual reality and implications for quantum consciousness, 2017). Its relationship to current interest in the Triple Helix model of innovation is also discussed separately (Reconciling triskelion and triple helix: a topological transformation with psychosocial implications? 2017). The following set of images are reproduced from that discussion.

Triskele/Triskelion (Celtic knot pattern and adaptation)	Transformation? via logical/systemic intermediaries	Triple helix (with adaptation to political systems)

Given the non-planar curvature required to meet the Borromean condition, it could then be argued that this offers an additional constraint on the enhanced subtlety appropriate to governance in an increasingly surreal era.

The Borromean condition suggests a fundamental simplification of the complexity represented by the Triskelion and and implied by any "Triple Helix". As it might be configured to meet that condition, does the triple helix then constitute the most "primitive" form of that helical condition -- a limit condition? One exploration of the relation of the Borromean condition to the double helix (which inspired the Triple Helix model) has been made (C. Mao, W. Sun and Nadrian Seeman. Assembly of Borromean rings from DNA. Nature, 386, 1997, 6621, pp. 137-138; see also Ned Seeman, DNA Borromean Rings; Kelly S. Chichak, et l, Molecular Borromean Rings, Science, 28 May 2004).

There does not appear to be any reference to a relation between the Borromean condition and the Triple Helix model of innovation -- nor to the extension of the triple form to a quadruple form (Simona Cavallini, et al, Using the Quadruple Helix Approach to Accelerate the Transfer of Research and Innovation Results to Regional Growth, Committee of the Regions of the European Union, 2016). Of especial relevance to the national debate in France which inspired this exploration, the fourth helix in that study is understood to be civil society -- the Gilets Jaunes? It is appropriate to note that such studies make no use of depictions other than those in 2D -- raising the question as to how misleading are 2D depictions in avoiding the challenge of comprehension indicated above.

Given the concern at this time with the ecological transition required by climate change (most notably in France), the use of two-dimensional depictions of quadruple and quintuple helix models to encompass such concerns is then also to be challenged as potentially misleading (Elias G. Carayannis, et al, The Quintuple Helix innovation model: global warming as a challenge and driver for innovation, Journal of Innovation and Entrepreneurship, 2012). One approach to the challenge of comprehension is discussed separately (Framing Cyclic Revolutionary Emergence of Opposing Symbols of Identity: Eppur si muove -- biomimetic embedding of N-tuple helices in spherical polyhedra, 2017).

Necessary cognitive twist: star symbols as bladed propellers -- for propulsion in 3D?

Misleading comprehension via 2D: Although interest in the Triple Helix model necessarily calls for the helical form, the restrictions of academic publishing typically force any depictions into a 2D format. Arguably this has cognitive implications with regard to comprehension of the nature of n-tuple helices. The issue can be highlighted in the case of the provocatively improbable structure known as the Discordian Mandala. This is depicted in 2D with the explanation offered by Wikipedia that some knot-theoretic links contain multiple Borromean rings configurations; one five-loop link of this type is used as a symbol in Discordianism, based on a depiction in the Principia Discordia.

However, as with the illusory depiction in 2D of the simplest Borromean example (as shown above), the question is how the form of such a Discordian Mandala might be depicted in 3D. This specific example framed a more general challenge explored separately with multiple images and animations in 3D (Concordian Mandala as a Symbolic Nexus: insights from dynamics of a pentagonal configuration of nonagons in 3D, 2016; Visualization in 3D of Dynamics of Toroidal Helical Coils: in quest of optimum designs for a Concordian Mandala, 2017).

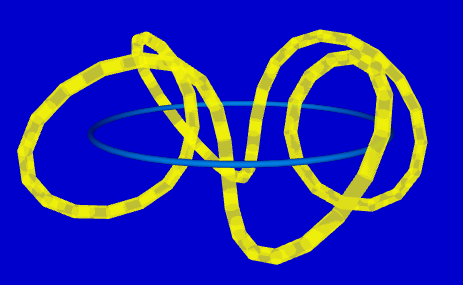
Requisite dynamics in 3D: The argument with respect to star symbols as requiring comprehension through their dynamics in 3D (introduced in the previous discussion) then calls for experimental depiction as shown below -- recognizing the constraints in presenting them in this mode. The point to be emphasized is that seen from "above" their helical nature is far from evident. Each is wrapped around a hidden torus -- of which only a pale blue tracing circle is an indication. This helical wrapping is far more evident in the second row of images offering a slanted view of each..

"Top" and "Side" views of helical loops with from 2 to 6 winds
Two	Three	Four	Five	Six

The argument here is that each symbol can be rotated on the central axis -- whether at a slow (and elegant) rate or at a speed recalling that of a propeller, namely the speed required for that rotation to exert a propulsive force (whether in air or in water). As seen from a perspective down the axis, however, it is far from evident why rotation should exert any force. The side view makes it clear that the windings (if completed as a surface, rather than left as an outline) function as vanes or propeller blades. As such they then recall the argument for a degree of equivalence with the propulsion achieved by 2-wnged birds or 4-winged insects -- transformed here into rotary motion of blades functioning as wings (as with the helicopter).

Slanting symbolic "blades" to a point: Of particular interest is how wings and blades are adjusted in response to various requirements of flight -- to be able to "cut it". This is in addition to the actual design of the blades, which is typically the focus of intense research. The visual renderings in 3D, as depicted above, are governed by design options regarding the dimensions of the helix. The formula for wrapping a helix around a torus to create the effect of blades is as follows.

Parameters for a helix of n winds wrapped around a torus of major radius R and minor radius r with t based on the number of sets of xyz coordinates.
(x coordinate)	(y coordinate)	(z coordinate)
x=(R+rcos(nt))cos(t)	y=(R+cos(nt))sin(t)	z= r(sin(nt))

The degree of slant to any "blade" is achieved by adjusting the relation between the major and minor radius of the implied torus. For the purpose of the above exercise, this was specified in terms of the golden ratio -- as offering an aesthetic result of potential significance to comprehension. Other effects can be achieved by making the major and minor radius virtually identical -- if the same, the helix would be effectively wrapped around a horn torus.

The golden ratio gives rise to symbolic stars which have seemingly rounded "points" (as shown above), whereas the horn torus would engender sharp points. There would appear to be an intermediary condition more closely corresponding to the manner in which symbolic stars are depicted in 2D. The sharper the apparent point, the more that point corresponds to a vertical line in 3D (along the missing dimension of a 2D depiction).

Further investigation of such proportions would determine how such variously "bladed symbols" would offer a third dimension to the star-symbols so readily misinterpreted in 2D: 2 (Tao?), 3 (Trinity?), 4 (NATO?), 5 (Pentagon?, Wu Xing?), 6 (Star of David?).

"Cognitive twist": Such misinterpretation can also be explored in terms of a requisite "cognitive twist" to achieve empowerment in psychosocial systems (Sphere eversion as guide to the cognitive twist of global introversion?, 2013; Toroidal configurations as fruitful loopery, 2015).

This can be understood as addressing the disconnect between "linear" strategic thinking in 2D, in contrast with a variously recognized need for "curvature" -- potentially consistent with any "global" understanding. Intriguingly, this is consistent with the need for spherical geometry to enable navigation (Global Psychosocial Implication in the Pentagramma Mirificum: clues from spherical geometry to "getting around" and circumnavigating imaginatively, 2015).

Stars and wheel spokes: Reflection on the number of "blades" required could usefully draw on the number required in propeller design. More generally it relates to the number of spokes required in a wheel for appropriate load distribution.

Of interest is the contrast in the number of spokes between recent designs for bicycle wheels (3, 4, 5 spokes) with the optimum number (15) found to be required for war chariots of the past. The cognitive organization of the octopus also merits consideration.

Direction of propulsion in a global context -- as enabled by configuration of symbolic stars

Direction, navigation and control: What "direction" of movement does a symbolic star imply in a global context: inward? outward? Where is the psychosocial system to go? Clearly some happily frame the response in terms of travelling "to the stars". For others it is some understanding of delocalization to globalization. There is also a degree of understanding of "inner space". A case can also be made for a degree of mirroring between outer and inner exploration (Global potential for living sustainably "outside-inside", 2013). Where indeed are we aspiring to go? Per ardua ad astra?

Greater clarification can be given to the response in terms of the requirement for navigation in 3D, as exemplified by control of spacecraft in orbit or control of vessels underwater. These clarify the need for propulsion in three mutually orthogonal directions. This is achieved by jets in space although use tends to be made of propellers underwater -- either set at right angles to each other, or capable of being oriented. Related solutions are evident with helicopters and drones.

Orthogonal dimensions: The argument here assumes that the three rings discussed in relation to the Borromean condition, so elegantly analyzed in relation to the three rings of the Divine Comedy (Saiber, 2013), bear some relation to any understanding of mutually orthogonal directions -- whatever their cognitive implications.

Given the constraint on the non-planar nature of a 3-ring Borromean condition, the question is then how three helices (as the alternative) might best be presented in a mutually orthogonal configuration. This is a challenge to representation in this web format, to topology (given issues of design optimization), to aesthetics, and to comprehension. Additionally, how might any such configuration be comprehended?

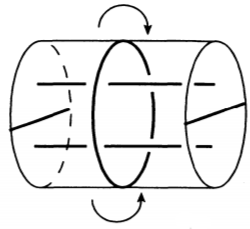
Multiple loops: As one approach to this possibility, the 3-looped helical representation above can be tentatively complemented by two other helical loops -- all in mutually orthogonal configuration. By allowing each helix to rotate, arguably they may move into or out of a Borromean condition -- or that specifically distinguished above as a (3,3)-torus link. This could then suggest that the Borromean condition is only met transiently -- an appropriate possibility in its own right. In this 3D virtual reality animation, the movement is not constrained -- loops can move through each other.

Rotation of 3-looped helices around mutually orthogonal Cartesian axes (animations)
Single loop (z-axis)	Two loops (x and z axes)	Three loops (x,y and z axes)

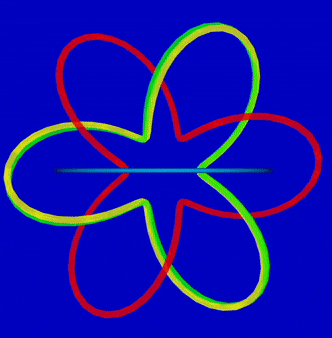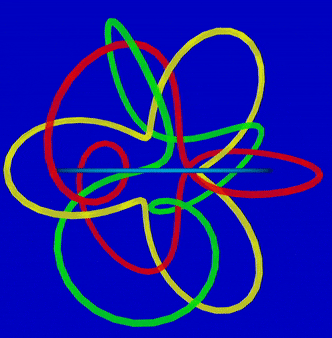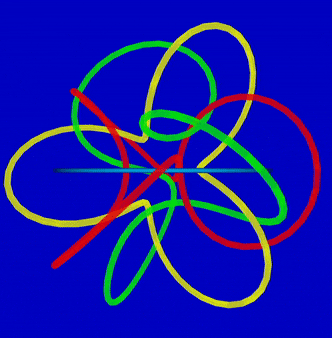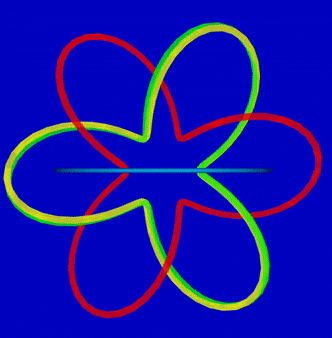Technomimicry? Beyond the wheel and the propeller, rotation of a single axis has been fundamental over the past century to the generation of electricity through dynamos and its use in electric motors. It is appropriate to ask whether the thinking of Nikola Tesla, as the key figure in that development, has implications for rotation around mutually orthogonal axes -- beyond that of operation of the gimbal, as illustrated in the previous discussion (Potential implications of alternation and rotation in psychosocial fields, 2014; Insight into global dynamics through Tesla's focus on the sphere, 2014).

Of particular interest are the potential consequences of rotation of such a psychososocial "magnetic field" on three axes rather than one. The possibilities are evident in the light of the heavy investment in toroidal reactors for nuclear fusion (Enactivating a Cognitive Fusion Reactor: Imaginal Transformation of Energy Resourcing (ITER-8), 2006).

Toroidal possibility: The following animation is suggestive in that respect. Of interest is the possible modification to parameters, notably the dimensions of the tori in relation to each other, and the rate of rotation of ech helix, especially at higher speed.

Three mutually orothogonal tori with a 3-loop helix moving over each of them (animation on right)
Video (mp4). Interactive virtual reality (x3d, wrl)

The configuration and dynamics recall those of the connections made and broken in a commutator and in the design of 3-phase electric motors.

Interlocking tori: Consideration can also be given to the relative movement of interlocked tori, as reprodiced below from a related discussion (Comprehension of Requisite Variety for Sustainable Psychosocial Dynamics: transforming a matrix classification onto intertwined tori, 2006).

Screen shots of a dynamic virtual reality model of intertwined tori (click on each variant to access and manipulate in 3D; in the free Cortona VRML viewer, right click for preferences to switch from/to the "wireframe" presentation)
	Red torus has a vortex (smoke ring) dynamic in the model
	Blue torus has a wheel-like dynamic in the model
VRML animation by Bob Burkhardt		X3D and VRML models (kindly developed by Sergey Bederov of Cortona3D).

Engaging globally with knots and riddles -- Gordian and otherwise

Gordian knot: The challenge of governance, whether global or otherwise, is articulated in terms of the traditional Gordian knot with which Alexander the Great was confronted. Recent examples include:

• John Jullens: The Gordian Knot of Global Economic Growth (Strategy-Business, 15 October 2013).
• Bernard Harborne and Bernhard Metz: Cutting through the Gordian Knot: analysis of conflict and violence (World Bank, 16 June 2015)
• Gabriela Lemus: Severing the 'Gordian Knot': defying conventional wisdom and reversing current trade policy (The Huffington Post, 2 June 2015)
• Reinhard Wagner: The Gordian Knot of Global Collaboration (International Project Management Organization, 22 January 2016)
• Pawel Opala and Krzysztof Rybinski: Gordian Knots of the 21st Century (2007).

The question is whether the structure of the knot, as a challenge to comprehension, can be explored more effectively, rather than simply treating it as a metaphor for "complexity", a discussed separately (Mapping grossness: Gordian knot of governance as a Discordian mandala? 2016).

Borromean rings as braid closure: Through metaphor, related characterizations can be explored in terms of the riddle of governance and the juggling of priorities (Global Governance as a Riddle: but is a solution the answer to the question? 2018; Governance as "juggling" -- Juggling as "governance": dynamics of braiding incommensurable insights for sustainable governance, 2018).

The latter discussion notes that juggling patterns can be understood in mathematical terms in the light of the theory of braids. That theory is critical to the theory of knots and links. Artists have been inspired by the Borromean ringgs as braids (Tom Verhoeff an Koos Verhoeff, Three Families of Mitered Borromean Ring Sculptures, Proceedings of Bridges 2015: Mathematics, Music, Art, Architecture, Culture, 2015).

Borromean rings, as a link of three unknotted loops (such that any two of the loops are unlinked) can therefore be understood as the closure of a braid, as discussed in some detail by Louis H. Kauffman (Knot Logic and Topological Quantum Computing with Majorana Fermions, arXiv, 2013).

Borromean rings as an ordered knot-set: Kauffman notes that as an ordered knot-set, the Borromean rings constitute a "scissors-paper-stone" pattern. Each component of the rings lies over one other component, in a cyclic pattern (left below).

Representations of Borromean rings (with Red surrounds blue; Blue surrounds green; Green surrounds red)
As an ordered knot-set	Toroidal version	3 Möbius strips (animation)
Reproduced from Kauffman (2013)	Reproduced from Kauffman (2006)	Video (mp4); Virtual reality (x3d; wrl)

Kauffman presented the same diagram in a communication to a less specialized audience, together with that in the centre above (The Borromean Rings: a tripartite topological relationship, Bridges, 2006). That in the centre is perhaps the most comprehensible representation of the rings. A highly provocative argument could of course be made that the link diagram (above left) may hold further significance through its correspondence to the most controversial symbol (Swastika as Dynamic Pattern Underlying Psychosocial Power Processes: implicate order of Knight's move game-playing sustaining creativity, exploitation and impunity, 2012). The Knight's move of chess, as explored, there forms a pattern curiously similar to that on the left above.

The "scissors-paper-stone" metaphor has been extended to 5-ring and 7-ring Borromean configurations (Marc Chamberland and Eugene A Herman, Rock-Paper-Scissors meets Borromean Rings, Grinnell College, 2014). As with the 3-ring game, the authors argue the case for 5-part and 7-part games with contrasting "weapons", noting that the number of such "games" has been extended to 13. Of relevance to this argument is whether the "weapons" are nations, as with the Group of 5 Permanent Members of the UN Security Council, the Five Eyes intelligence alliance, the Group of 7, or sets of mutually overriding strategic priorities of governance. Do 3-fold groups invite similar insight (Trilateral Commission, Trilateral Cooperation Secretariat)? What then of the viability of any cartel or crime ring, most notably triads?

Borromean Rings understood as weapons in a "Rock-Paper-Scissors" Game
The Game	Discordian mandala	5-fold Borromean rings	7-fold Borromean rings
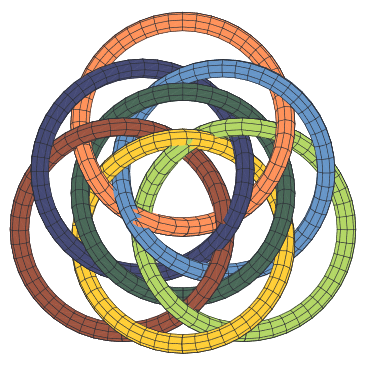Reproduced from Wikipedia		Reproduced from Chanberland and Herman (2013)

Borromean rings and "unknotting"? Such patterns merit exploration in relation to the Reidemeister moves of knot theory, as mentioned by Kauffman (2013). It is surprising to note that the relation between the Borromean rings and the Reidemeister moves has been primarily indicated through music (Robin Hayward and Christopher Williams, Reidemeister Move plays Borromean Rings, Corvo Records, 2014). This recalls the effort to use song as a mnemonic aid to comprehension of the pattern of metabolic pathways (Harold Baum, Biochemists' Song Book, 1995; The Biochemists' Songbook MP3 Files).

Interest in Borromean rings in knot theory has an intiguing relation to understanding of what is termed the unknot (namely a circle). Intertwined as they are, and as depicted, the three rings can be understood as an entanglement of unknots. However this evokes questions such as Why is the unknotting number of Borromean rings 1? (Mathematics, 29 May 2017).

To the extent that complexity may be a consequence of distorted perception of wholeness, a further question lies in the challenge of recognizing whether what is (potentially erroneously) perceived as knotted is in fact an unknot. The answer is explored in relation to Graham's number, reputed as the largest number to have ever shown up in any mathematical proof (John Baez, An Upper Bound on Reidemeister Moves, Azimuth, 9 March 2018; Alexander Coward and Marc Lackenby, An upper bound on Reidemeister moves, American Journal of Mathematics,  136, 2014, pp. 1023-1066).

Are proposals for dialogue, as is currently the case in France with respect to the Grand Débat, to be usefully reframed as an "unknotting" process? Problematic however is the charactristic of many dialogues in which the degree of knotting in fact increases rather than decreases -- despite purported aspirations to achieve an unknotted condition. Such considerations raise the question as to whether agreements may take the form of particular knots -- of which various knot tables and knot tabulation may offer an indication, notably when ordered by number of crossings and links (List of prime knots, Wikipedia).

Knot tables as an indication of conditions of dialogue and degrees of agreement?
Prime knots up to seven crossings; labeled with Alexander-Briggs notation	All simple knots admitting a projection on the plane with 9 or fewer double points
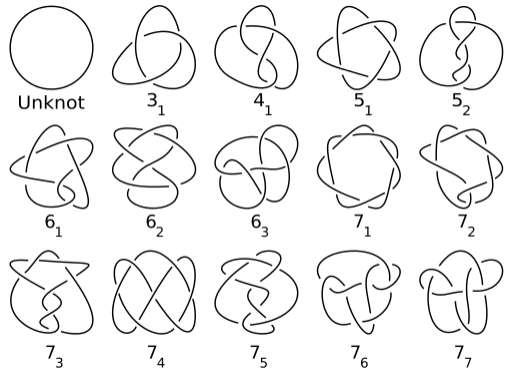Reproduced from Wikipedia	Reproduced from knot table (Encyclopedia of Mathematics)

Of potential relevance to knotting and unknotting are explorations of geometric moves on knots, as described by Junghwan Parl (Milnor's Triple Linking Number and Derivates of Genus Three Knots, arXiv, 30 March 2016):

Double Borromean rings insertion move

Reproduced from Park (2016)

There is a further intriguing possibility that considerations of patterns of knotting may be relatted to those of patterns of knitting -- which, at least metaphorically, is valued in terms of "knitting" relations together (Evelyn Lamb, Knotted Needles Make Knitted Knots, Scientific American, 26 February 2014; Borromean Cubes, Wooly Thoughts). The feasibility of the use of the mysterious Roman dodecahedron for knitting has been variously demonstrated (Knitting with the Roman dodecahedron, YouTube, 1 July 2014; Martin Hallett, Has The Roman Dodecahedron Mystery Been Solved? YouTube, 3 June 2014). The possibility is somewhat reminiscent of the use of qhipu as the mode of communication in the Andean civilization, a pattern of knots now in process of decoding by the Harvard Khipu Database Project (String, and Knot, Theory of Inca Writing, The New York Times, 12 August 2003).

Borromean rings and quantum entanglement: The pattern above enables Kauffman to note the relation to the Greenberger-Horne-Zeilinger state, namely a certain type of entangled quantum state that involves at least three subsystems (particles). Cting P. K. Aravind (Borromean entanglement of the GHZ state, 1997), Kauffman notes:

The Borromean rings are entangled, but any two of them are unentangled. In this sense the Borromean rings are analogous to the GHZ state... Measurement in any factor of the GHZ yields an unentangled state. Aravind points out that this property is basis dependent. We point out that there are states whose entanglement after an measurement is a matter of probability (via quantum amplitudes).... New ways to use link diagrams must be invented to map the properties of such states. [emphasis in original]

The University of Bergen had a website dedicated to Radioactive Nuclear Beam Theory and the implication of the Borromean rings. The work has focused on few-body theory for light halo-like Borromean nuclei (Jan S. Vaagen, et al., Borromean Halo Nuclei: Continuum Structures and Reactions; M. V. Zhukov, et al, Bound State Properties of Borromean Halo Nuclei, 1993; C. A. Bertulani, et al, Geometry of Borromean Halo Nuclei, 2007). The 3-body quantum analogue is one where the 3-body system is bound, but none of the 2-body subsystems are bound.

Framing fundamental research in terms of "few-body" theory is suggestive of the need for a similar framing in the psychosocial sciences -- "few-category" theory or "few-concept" theory. Of potential relevance are the application of quantum insights to the social sciences by (Alexander Wendt, Quantum Mind and Social Science: unifying physical and social ontology, 2015), as discussed separately (On being "walking wave functions" in terms of quantum consciousness? 2017).

There is potentially great irony to the fundamental symbolic importance traditionally associated with the Sun, now that research is discovering the role of light halo-like Borromean nuclei in solar physics -- and more generally in astrophysics (B. V. Danilin, et al. Cluster models of light nuclei and the method of hyperspherical harmonics: successes and challenges, Physics of Atomic Nuclei, 72, 2009, 8, pp. 1272-1284).

Hyperbolic implication of Borromean rings: The complement of Borromean rings is octahedral and hence embeds geodesically in a finite volume hyperbolic four-manifold. The Borromean rings complement is tessellated into two ideal octahedra (B. Martelli:, Hyperbolic 3-manifolds that embed geodesically, arXiv:1510.06325, 2015). All known examples of geodesically bounding hyperbolic link complements are arithmetic and have each of their respective components unknotted. From that perspective the Borromean rings complement is a thrice punctured torus bundle by specifying automorphism of the surfaces in question (Christof Menzel, The Whitehead Link Complement and the Borromean Rings Complement are Torus Bundles, 11 June 1999).

Borromean rings as an Euclidean orbifold: sequence of transformations of an Euclidean cube into a hyberbolic orbifold
Adapted from D. Cooper, Orbifolds. Project Euclid, ?2000

Soap films on Borromean rings: An unusual representation of Borromean rings is based on their support for many distinctive soap films, as presented by Ken Brakke (Soap films on Borromean rings) The author indicates 14, of which a selection is presented below, although others are possible. The images were made with his Surface Evolver program. The films are noted as obeying Plateau's Laws of Soapfilms, namely that the three films meet along a curve at equal angles, and three such triple curves meet at a "tetrahedral point" at equal angles.

Selection of soap films on Borromean rings

Reproduced from website of Ken Brakke

Laws of form: Also of relevance is George Spencer-Brown's Laws of Form (1969), as variously discussed by Kauffman (Laws of Form: an exploration in mathematics and foundations). As Kauffman (2013) notes in a preamble to his discussion of Borromean rings:

Laws of Form begins with the following statement. We take as given the idea of a distinction and the idea of an indication, and that it is not possible to make an indication without drawing a distinction. We take therefore the form of distinction for the form. Then the author makes the following two statements (laws):

1. The value of a call made again is the value of the call.
2. The value of a crossing made again is not the value of the crossing

Understood in the light of the Laws of Form as constituting category boundaries, the Borromean rings (separately and together) raise the question as how such boundedness should be understood as sustaining a distinction -- and the nature of the misunderstanding when this is not appreciated. The Borromean rings are a form of (re)presentation.

As discussed separately (Form, geometry, pattern and dimensionality, 2007), the focus of Michael Schiltz (Form and Medium: a mathematical reconstruction, Image [&] Narrative, 6, 2003) follows from that of the "calculus of indications" of Laws of Form. Schiltz notes that form/medium is the image for systemic connectivity and concatenation, as described by Humberto Maturana and Francesco Varela. For Schiltz, the notion of "space" is the key to reflexivity appropriate to any discussion of form and medium, citing Spencer-Brown as follows:

In all mathematics it becomes apparent, at some stage, that we have for some time been following a rule without being aware of it. This might be described as the use of a covert convention. [… Its] use can be considered as the presence of an arrangement in the absence of an agreement. For example, in the statement and theorem.... it is arranged (although not agreed) that we shall write on a plane surface. If we write on the surface of a torus the theorem is not true […] The fact that men have for centuries used a plane surface for writing means that, at this point in the text, both author and reader are ready to be conned into the assumption of a plane writing surface without question. But, like any other assumption, it is not unquestionable, and the fact that we can question it here means that we can question it elsewhere.

Conversation theory, actor interaction and boundaries

Knotted arguments? The nexus of threads above could be considered a knot in its own right. Whether in fact it is an unknot is another matter. To what extent should the "links" made between elements of an argument (as in this text) be understood as constituting a "knot" -- given the manner in which "links" are a major feature of knot theory? Understood in this way, could the preoccupations of citation analysis benefit from insights from knot theory in order to clarify contributions to a thematic domain?

Potentially more intriguing are the implications of an exercise portraying Borromean rings as composed of 3 Möbius strips (see animation above), given the challenge to insideness versus outsideness widely associated with such single-sided strips.

A previous exercise explored the association of a Möbius strip with a torus in relation to animation of toroidal knots (Eliciting the "pattern that connects" via "bull"?, 2017). An earlier exercise used an animation of three mutually orthogonal Möbius strips (as above) to frame the experiential significance of begging (Mapping the cognitive dynamics of the begging moment, 2015). Is "begging", more generally understood, now fundamental to the relation between the variously disempowered and disenfranchised and the "international community" and its elites?

Beyond dispute? With agreement commonly associated with a circle, more intriguing is the question of whether the knot-like nature of apparent disagreement obscures less evident agreement -- in the sense in which it conceals an unknot and the possibility of any superordinate frame (Using Disagreements for Superordinate Frame Configuration, 1992). Is it possible that the Gordian knot of global governance could be an unknot -- considered otherwise?

The literature on knot theory and the Borromean rings is variously associated with that on polyhedra. It is therefore interesting to note the probable relationship to the field of oppositional logic and the associated oppositional geometry, given its use of polyehdral models as discussed separately (Oppositional Logic as Comprehensible Key to Sustainable Democracy: configuring patterns of anti-otherness, 2018).

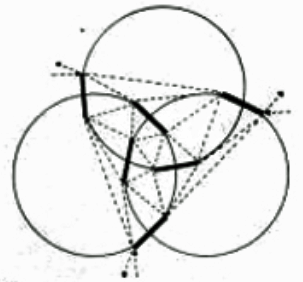
Of related interest is the work of management cybernetician Stafford Beer (Beyond Dispute: the invention of team syntegrity, 1994) which explored the use of a icosahedral model especially in the form of a tensegrity. This integrates the balancing dynamics between non-compressible ("non-negotiable") and flexible elements in group discourse, as suggested with respect to a UN Earth Summit (Configuring Globally and Contending Locally: shaping the global network of local bargains by decoding and mapping Earth Summit inter-sectoral issues, 1992). In the light of that focus, it is somewhat ironic to note that if the vertices of an icosahedron are connected to form mutually perpendicular golden rectangles, the boundaries of the rectangles form Borromean rings.

Borromean rings formed by golden rectangles of an icosahedron

Reproduced from Wikipedia

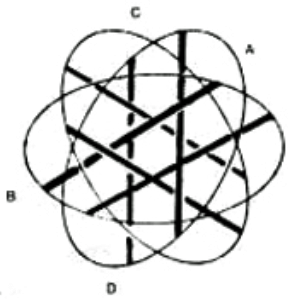
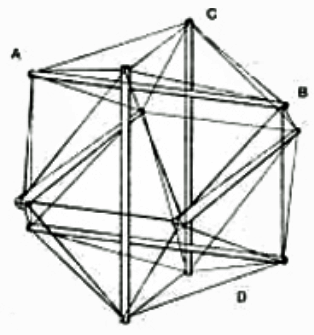
With respect to the earlier mention of Venn diagrams in relation to Borromean rings, there is a case for exploring stages in the transformation into tenesgrity structures as illustrated from a separate argument (Implementing Principles by Balancing Configurations of Functions: a tensegrity organization approach, 1979)

Transformation of a 2D Venn diagram representation into a 3D tensegrity

Such illustrations highlight the potential cognitive significance of Borromean rings in relation to the great circles by which symmetrical polyhedral forms may be framed, and the implications for spherical geometry (Beyond dispute in 5-dimensional space: Pentagramma Mirificum? 2015).

Conversation theory: Of particular relevance to this argument has been the manner in which conversation theory has been developed (Gordon Pask and Gerard de Zeeuw, Interactions of Actors: theory and some applications, 1992), with reference to the work of Beer (1994), notably as described by Nick Green (Axioms from Interaction of Actors Theory, Kybernetes, 33, 2004) with respect to triplets of concept resonnce:

There are two parts to an interacting participant or Actor. First the P-individual which is a dynamic, productive and incidentally reproductive, adaptive, evolving and learning collection or entailment mesh of concepts. Dawkins' memes (Dawkins, 1976) are a restricted form of P- individual. Second the M-individual which is a mechanical or biological medium e.g. a computer, a brain or a star, which supports the P-Individual and the strains its concepts produce....

The closed toroidal processes which comprise the concepts of P-individuals exist as stable triples in which any pair is analogous to the other and distinguished by the third. Any two concepts may generate the third because of their resonant similarities and differences. The resonance produced by an incident field produces an output radiative field. Knot theory was a matter of some concern to Pask. He coined the term "tapestry" making his entailment mesh structure of the concepts of a participant coherent with knot theory. Whilst loops are always permitted, indeed are the nature of concepts, the intersections of C[onversation] T[heory] become crossings in I[nteraction of] Actors Theory] where crossings up and down with loops define knots. The crossing up or down rule of the knots or links was not decided but a recursive and nested Borromean form seemed most likely. This seems coherent with the superstring theory interpretation of force. (pp. 1433-1435)

Pask's Stable Concept Triple linked as a Borromean Ring Orthogonal form of the Borromean link-isometric view. A putative model of Continuity, the equilibrium of void and not-void, around a void.

Reproduced from Nick Green (Axioms from Interactions of Actors Theory, 2004)

It is appropriate to note the collaborative effort of Beer and Pask to apply their early insights to the experimental reframing of a conference in 1979 of the Society for General Systems Research, as separately documented (Metaconferencing: discovering people / viewpoint networks in conferences, 1980; Participant Interaction Messaging: improving the conference process, 1980). Despite the subsequent development of their insights, as indicated above, it is unfortunately the case that it has proved impossible to apply them to any conferences of significance (History of Participant Interaction Messaging, 1979 to 1995, 2007). This is the case even though groupware technology, social network analysis and dialogue analysis have become remarkably sophisticated in the internet era.

As an example, in relation to the (forthcoming) Grand Débat national in France, evoked by the Gilets Jaunes crisis for democratic governance, there are no calls for the use of such insights to achieve a higher order of coherence and reconciliation -- despite an anticipated "dénouement" to the crisis.

Drama, denouement and cycles: Given the interest of artists in Borromean rings, it might be expected that the of drama on a denouement would have elicited interest in the understanding of unknotting in knot theory -- and the role of the unknot, or possibly the rings, as the culmination of that process. This could be seen as especially relevant given current interest in narrative in policy-making (Elizabeth Shanahan, et al, Denouement?: The Science of Stories, 2014; E. King and R. Wells, Framing the Iraq War Endgame: War's Denouement in an Age of Terror, 2009). The sense of the knotted and the unknotted thread in tragedy is understood to go back to Aristotle's Poetics, as described by J. Hillis Miller (The Problematic of Ending in Narrative, Nineteenth-Century Fiction, 33, 1978, 1)

Only a passing mention of this understanding was found (M. Pizzato, Ghosts of Theatre and Cinema in the Brain, 2006, p. 271). The possibility of such recognition in temporal terms is better framed by Ron Jenkins (Mathematical Topology and Gordian Narrative Structure: "Tristram Shandy", Mosaic: An Interdisciplinary Critical Journal, 25, 1992, 1, pp. 13-28). This raises the question as to whether knots, and the Borromean rings, could be understood in terms of temporal cycles as explored by social cycle theory. As variously recognized, are any such cycles to be recognized as configured in a Borromean condition -- especially when of a dissimilar but complementary nature? (List of cycles, Wikipedia).

Potentially more challenging is the sene in which the many calls for peace and unity by world leaders may require an appreciation of the Borromean condition (despite its illusory presentation in 2D) rather than the illusory requirement of everthing interlocking with everything (as in the (3,3)-torus link depicted above).

Knots in psychoanalysis: Missing from such elegantly sophisticated considerations of knots is any reflection whatsoever by the "natural sciences" on their relevance to the Gordian Knot of govenance at this time. Curiously, there is seemingly complete indifference within that framework to the challenge of how insights from knot theory are perceived or comprehended.

Considerable interest in knots and knot theory is characteristic of the psychoanalysts Jacques Lacan and R. D. Laing. Why the topological considerations of knot theory have been important to psychoanalysis has been variously clarified (Bruno de Florence, Lacan and Topology, Lacanian Works, 8 October 2011; Owen Hewitson, From the Bridges of KÖnigsberg: why topology matters in psychoanalysis, LacanOnline.com, 9 January 2015). The latter notes that Lacan is recognized as having lived on "Planet Borromeo".

As indicated in the Encyclopedia of Lacanian Psychoanalysis, Lacan explored the use of the Borromean knot as, among other things, a way of illustrating the interdependence of the three orders of the real, the symbolic and the imaginary -- a means of comprehending what it is that these three orders have in common. A depiction of the Borromean knot is used as the logo of that Encyclopedia.

In exploring the double-bind, Laing framed "going mad" in terms of an "incompatible knot" (Knots, 1970). With respect to global governance at this time, there is no lack of reference to the sense that the "world is going mad", notably through characterization of its leadership. This understanding is expressed otherwise in terms of the surreal (Surreal nature of current global governance as experienced, 2016).

One psychologist, having initially framed his interest in terms of the Möbius strip (Steven M. Rosen, The Moebius Seed: a visionary novel of planetary transformation, 1985), has then proceeded to articulate wider concerns from a topological perspective (Topologies of the Flesh, 2006; Dimensions of Apeiron: a topological phenomenology of space, time and individuation, 2004; Dreams, Death, Rebirth: a multimedia topological odyssey into alchemy's hidden dimensions, 2014). Without any reference to knot theory or Borromean rings, these have been framed by the Klein bottle -- with which the Möbius strip is so closely related.

Paradoxical nature of boundaries: It could be asked whether the widely noted paradoxes of the Möbius strip and the Klein bottle offer clues to richer ways of engaging cognitively with the Borromean rings. Of some relevance to climate change concerns, separately it is argued that the traditional symbolism of the Ouroboros holds some of the dimensions of both forms (Global Conveyor, Rainbow Serpent and Ouroboros, 2007; Cognitive Osmosis in a Knowledge-based Civilization: interface challenge of inside-outside, insight-outsight, information-outformation, 2017).

"Boundaries" are fundamental to the identity of countries, disciplines and groups. They are of primary significance to engendering conflict -- as is currently evident in relation to migation. How might "crossing the line" be otherwise understood, in the light of the Möbius strip for example? (R. Jeffrey Smith, Crossing the Line at Odd Times: China-India Border Disputes, Foreign Policy, 16 October 2014; Jim Murphy, Crossing the Line, Public Policy Research, 18, 2011, 1; Jordan Schachtel, Crossing the line from non-interventionist to foreign propagandist, Conservative Review, 17 April 2018).

Dynamics implied by circles and rings: As the simplest boundary, symptomatic of coherence and integrity, a circular ring can be understood as implying the possibility of various distinct dynamics -- potentially of cognitive significance in relation to any boundary function, beyond static understanding of the form. Such dynamics are recognized to a degree in their use as symbols, most notably the ring (Engaging with Globality -- through cognitive lines, circlets, crowns or holes, 2009). The implication is of particular interest in relation to rings configured in a Borromean condition -- typically considered as static, despite their potential transformations through the pattern of knots:

• ring rotation -- rotary motion, as with any spinning wheel
• ring involution/evolution -- a vortex (smoke ring) motion, as associated with annular muscles
• ring spinning -- as with a coin spinning on a point on the circumference, or inverting ("flipping") the coin to expose the other side

Understood as a torus, the ring may also frame movement around it, as with the circular motion of planets, or the movement of plasma in a toroidal fusion reactor. The Ouroboros is a traditional symbol of this, with dynamics inviting suggestive animation (Experimental animations in 3D of the ouroboros pattern, 2017).

To the extent that the Möbius strip and the Klein bottle variously embody circularity in a manner to exemplify paradox, it could be asked -- especially in relation to the latter -- how they might be seen as embodying some kind of transformation of the Borromean condition, dynamically understood. In topological terms, with respect to the circular cross-section, there would appear to be various transition points (possibly three?) in traversing the Klein bottle, each invoving a transformation of the ring. The question is how those dynamics might be experienced as transforming the cognitive significance of what is bounded by the ring -- through redefining the nature of the boundary (Intercourse with Globality through Enacting a Klein bottle, 2009).

Dante's three giri: As implied above, the interdisciplinary study by Arielle Saiber and Aba Mbirika (The Three Giri of Paradiso XXXIII. Dante Studies, 131, 2013) is remarkable for the balance it brings to aspects of the issue discussed here. It interweaves consideration of the Borromean rings with extensive commentary on relevant knot theory and topology. It is appropriate to note that those indicated in that study who have explored the matter naturally include a number inspired by the theological implications. If nothing else, it is valuable as a review of the ways in which the reconciliation of three incommensurables may be most fruitfully considered in the light of thinking in he past.

It engages with Dante's poetic insights into Christianity's greatest paradoxical mystery of three-in-one / one-in-three. In this sense it could be understood as a study in "topological theology" or "theological topology". Those implications reinforce the case for more extensive research in mathematical theology, as separately argued (Mathematical Theology: Future Science of Confidence in Belief -- self-reflexive global eframing to enable faith-based governance, 2011).

As the authors summarize:

... we analyze one particularly suggestive arrangement for the giri: that of three intertwined circles in a triangular format. Of the many permutations of this figure, we isolate two variations -- a Brunnian link commonly called the Borromean rings and a (3,3)-torus link -- to show how they more than any other possible arrangement offer unique mathematical, aesthetic, and metaphoric properties that resonate with many of the qualities of the Trinity Dante allusively described in Paradiso 33. We propose these as a possible configuration, rich with mystery in themselves, out of a number of Trinitarian models that Dante knew and contemplated. (p. 239)

Comprehension of elusive connectivity and coherence for sustainable governance

It is appropriate to recall that the focus here on the interlinking of three rings emerged from consideration of issues of seemingly incommensurable three-foldness in governance (Triangulation of Incommensurable Concepts for Global Configuration, 2011). This is most notably evident between the mindsets, priorities and language of right-wing, left-wing and centre in political systems, or between academia, business and government, as reflected in the current preoccupation of the Triple Helix model. In the language of classical mechanics it could be understood as the challenging dynamics of the three-body problem and its integrative comprehension.

The traditional theological preoccupation with any trinity can then be understood as another language through which this cognitive challenge to coherence has been explored. One insightful clarification of the challenge is itself articulated through a triangle of colours in mathematicaal terms by Ron Atkin (

Implications of polygonal configurations for comprehension of complexity: Of potential relevance to this argument is the attention given to developing an appreciation of nine-foldness in children, as described by Sheryl Nichols Morris (Nine: Observing Nine with Young Children (SNAP: Scaffolding for Numerical Synapses: Awakening Curiosity in the Numbers One to Ten. 2013).

The challenge of acquiring such insight -- beyond the binary -- is remarkably articulated using a triangle, from the mathematical perspective of q-analysis by Ron Atkin (Multidimensional Man; can man live in 3-dimensional space?, 1981). Atkin illustrates the challenge of comprehension in relation to experience constrained "within" the geometry of a triangle -- especially with regard to the perspective necessary to comprehend the geometry of the triangle as a whole, as separately summarized (Comprehension: Social organization determined by incommunicability of insights).

The constraints are a consequence of being trapped in a form of tunnel vision "within" the lines (of argument) configured as communication traffic around the point (especially when the lines are constructed as cylinders). This inhibits the acquisition of any sense of perspective. The cognitive significance of this approach is well-illustrated by visual sensitivity to colours resulting from the three primary hues (red, green and blue). These may be represented on a simple triangle. Here the vertices (O-simplexes) represent the primary hues, the sides are twofold combinations (1-simplexes), and the combination of the three hues makes the central white (2-simplex).

Challenge of recognizing a higher-order perspective schematic used by Ron Atkin
	0-dimension vision: Red, Green or Blue 1-dimension vision: Yellow (=Red/Green) Purple (=Red/Blue); or Turquoise (=Blue/Green) 2-dimension vision: White (=Red/Green/Blue)

The relevance of q-analysis has been more recently clarified (Konstantin Y. Degtiarev, Q-Analysis and Human Mental Models: a conceptual framework for complexity estimate of simplicial complex in psychological space. 2011; Jacky Legrand, How far can Q-analysis go into social systems understanding?).

Divinity -- as a metaphor? The Saiber study (2013) has the merit of framing the quest with the respect for subtlety associated with divinity -- despite the various topological formalisms to which reference is made. As a quest, the sustainability for "governance" implied by that comprehension (as it might be most generally understood) can similarly be compared with that for the Holy Grail (In Quest of Sustainability as Holy Grail of Global Governance, 2011; Larry Miller, Sustainability: the New Holy Grail, Political Christian, 29 December 2010). Aside from the traditionally articulated dangers of "grasping" and seeking to possess it, that form lends itself to comprehension through catastrophe theory (Interrelating Cognitive Catastrophes in a Grail-chalice Proto-model, 2006). Fundamentally the issue may be one of perspective and perception.

As noted by Saiber (2013), regarding perception of the three "giri" by the Pilgrim in Dante's Paradisio:

It (or "they", as we will consider below: parvemi or parvermi ?) appear in the form of (or looked like to him) three giri (circles/discs, spheres/balls, tori, cylinders, spirals, ellipsoids, or other round things?), di tre colori (each a different color, or each containing three colors?), and d'una contenenza (all the same size, all occupying a single space, all contained within a single space, and/or all of the same substance?). One of the giri appears as a reflection of another, come iri da iri (resembling a double rainbow, or merely an analogy for generation?), and the third giro appears as a flame (parea foco) breathed or breathed forth in equal measure (si spiri) by the first two (quinci e quindi). (pp. 207-8)

The definition of the form itself is elusive:

What begins to become apparent is that the actual shape of the giri could be one or more of these "roundnesses" simultaneously, especially if the giri were in motion. A ring (circle) spinning on its edge, for example, could look like a sphere revolving about its center, like a wheel or a disc. And depending on the figures' configuration, other "roundnesses"' could emerge to the eye (p. 242)

Dynamics? Their movement is a factor, raising the question as to the possible nature of that movement:

If we read Dante's giri as "roundnesses in motion", what are the possible ways in which roundnesses could move? The poet certainly does not say anything specific about motion; nor does he leave any clues. Each giro , if flat like a circle or disc, could be turning around its own central axis like a pinwheel or frisbee; or it could be spinning on an edge like a coin dropped on a table. If the giri are spheres, then they could look like tennis balls with topspin.... Furthermore, the giri could also be partaking of multiple types of circular motion.... Many medieval theologians held that God's thought moved circularly (human thought linearly, and angelic thought in a spiral) and that to contemplate God we too needed to set our mind moving in a circle. (pp. 245-7) [emphasis added]

There is a provocative irony to framing the spiral as characteristic of angelic thought, given the current secular preoccupation with the Triple Helix model of innovation (Reality of the demonic and the angelic by comparison with secular understanding of existence, 2016).

Three-dimensionality? Little consideration has however been traditionally given to comprehension in three-dimensions, although it is noted that one the most sustained meditations on circular geometry as a key to understanding God is the work of Nicholas of Cusa (David Albertson, Mathematical Theologies: Nicholas of Cusa and the Legacy of Thierry of Chartres, 2014, especially pp. 243-52), As the study notes:

Most commentaries on the giri's configuration have imagined them to be arranged in a planar format. Only a few have theorized how the giri could, instead, be arranged using spherical geometry.... It is not clear, in fact, from Dante's description of the giri how he intended them to be linked; but they had to be united/unified in some way.... The most commonly-imagined arrangement of the giri, however, has been of three intertwined circles, which as knots, are actually occupying three dimensions, but when seen face on, can seem flat....The final section of this study is a topological and combinatorial analysis of the layout of three intertwining circles we find particularly compelling vis-a-vis Dante's description of the Trinity: the round figures arranged in a triangular format with rotational and reflective symmetry. (pp. 255-260) [emphasis added]

Comprehension? Appropriate to this argument, the analysis uses "vertigo" to name the challenge to comprehension:

When we think of all the possible ways three circles could be linked, as we have done so far in this essay, a kind of vertigo begins to set in. If we narrow our focus to just a single arrangement -- that of three, linked circles in a triangular format, it turns out that there is a limit to how many ways they could be configured: there are only sixty-four. These sixty-four patterns of "links", as topologists call them, can be sorted into five categories. Of these five categories, there are two... that provide us with particularly intriguing models for Dante's Trinity: the Borromean rings and the (3,3)-torus link (p. 260) [emphasis added; see overlapping circles grid]

Transience? Given both the potential significance of movement and the 64 patterns, there is a case for recognizing that, rather than being "permanent", the elusive unity implied may involve a high degree of transience, as extensively explored in the argument of Raymond Tallis (Of Time and Lamentation: reflections on transience, 2017).

The pattern of 64 is of course characteristic of the Chinese classic, the I Ching, in which such completion is but one phase in a cycle (Transformation Metaphors: derived experimentally from the Chinese Book of Changes (I Ching) for sustainable dialogue, vision, conferencing, policy, network, community and lifestyle, 1997). It is in this sense that one animation to be explored would have the three linked circles cycling through all 64 phases. Aspects of the case are presented separately (Reframing the Dynamics of Engaging with Otherness: triadic correspondences between Topology, Kama Sutra and I Ching, 2011).

Unrecognized reminder of globality from the focus of ball games

The considerations above are relatively abstruse -- as with the cognitive implications of "globalization", "unity" and "integration" to which reference is so frequently made, There is however considerable irony to the trace of such forms to be found in the designs of balls used in the most common ball games. Although "global" may be an elusive concept for many, if not most, this is no hindrance to the attraction of a ball and its use in ball games. There is a sense in which one is effectively mirrored in the other -- and the pleasure derived from kicking, hitting or throwing a ball, and possibly of catching it, which (in a condution of global crisis) might otherwise be considered perverse.

The forms discussed above are evident to a degree in the patterns of stitching and seams in the common association football, the baseball cover, and the tennis ball -- and presumably in the possible design and manufacture of balls for other sports:

• Lise Sissler: Advanced Modelling and Design of a Tennis Ball (Loughborough University, 2011)
• Richard Thompson: Designing a Baseball Cover (The College Mathematics Journal, 29, 1998, 1)
• Batdelger Doljin and Franz Konstantin Fuss: Development of a smart cricket ball for advanced performance analysis of bowling (Procedia Technology, 20, 2015, pp. 133-137)
• Dimple pattern and the placement structure on the spherical surface of the golf ball, (US Patent, 1993).

Tennis ball	Soccer ball (with icosahedron)		Rugby football (with oblate and prolate spheroids)
Images reproduced from Wikipedia

The Sissler study of tennis ball prototypes and their characteristics is especially suggestive in the light of the argument above. It includes the following images.

Tennis ball prototypes (studied in relation to spherical polyhedra)

Reproduced from Sissler (2015)

Parametrization of baseball seam (views from z-axis and y-axis)	Yin-Yang construction curve design for the baseball seam (leading to patent by Jackson in the 1860s)
R. Thompson, Designing a Baseball Cover (1998)	Cameron Browne, Tanji Variations (2007)

There are studies of preferences for gaming styles (especially video games), although seemingly not for preferences for ball-games, especially in terms of the balls used (Bart Stewart, Personality And Play Styles: a unified model, Gamasutra, 1 September 2011; Allen R McConnell, The Psychology of Sports Fandom, Psychology Today, 13 July 2015; Eric Simons, The psychology of why sports fans see their teams as extensions of themselves, The Washington Post, 30 January 2015). Are such preferences -- through the form of the ball and how it is played -- in some way correlated with cognitive engagement with any "global" dimension and the opportunities for "uplift" it offers?

To what extent do the constraints on ball design and manufacture reflect constraints on the conception, design and implementation of global strategies, as discussed separately (Nature of the "ball" in game-playing and governance? 2016). Especially intriguing is the possibility that preferred ball-games, with their respective ball designs, may reflect preferred comprehension of global strategies. From that perspective the design of the rugby football, as a major preference of some Western countries (notably the USA), exemplifies a very particular preference for global polarization in terms of an oblate/prolate spheroid.

Is the nature of globality, as it may be comprehended, essentially associated with the dynamics of some form of play -- and cannot be effectively understood otherwise? Aspects of this question are discussed separately (Playfully Changing the Prevailing Climate of Opinion: climate change as focal metaphor of effective global governance, 2005; Humour and Play-Fullness: essential integrative processes in governance, religion and transdisciplinarity, 2005; Enacting Transformative Integral Thinking through Playful Elegance, 2010). Reference to Dante's Divine Comedy is then especially appropriate,

It is intriguing to note the heavy investment and identification in two-team ball-games, whose dynamics are well-recognized as being echoed in the dynamics of political and military system -- for which they offer formative experience. Despite the evident challenge of any third actor, every effort is made to reframe the dynamic n binary terms -- with every effort by the dominant party to suppress or eradicate the other. This does not bode well for the Triple Helix model or any analogue to it (right-wing, left-wing, centre). There is little appreciation of 3-sided games, despite one innovation as 3-sided football. Given the accepted sexist distinction made between male and female ball-games, despite the vigorous challenge to such distinctions in other contexts, the possibility of 4-sided football -- with male and female teams playing "across" each other -- has not been explored (Destabilizing Multipolar Society through Binary Decision-making: alternatives to "2-stroke democracy" suggested by 4-sided ball games, 2016).

There is however an additional irony in that technology is now widely used to map and explain visually the dynamics of the evolution of any ball-game -- especially in terms of movement of the ball between players and teams ("passing patterns"). There seems to be no apparent effort to map the dynamics of any dialogue, whether in parliamentary assemblies or conference panel sessions. The question as to why this not done -- given the purportedly desperate quest for effective dialogue -- has become far more relevant than the fact that it is not done. The question is especially pertinent in a country like France, which is in process of organizing a Grand Debat regarding its governance. The current regume in France lays specific claim to having a deep commitment to development and adoption of digital technology.

The potentially fundamental nature of such questions is evident in the Cloverleaf model of tRNA. The tracery on the tennis ball, and its relation to the forms discussed above, is especially evident when interlocking of the implied tori is rendered evident

Constraints on comprehension and communication: technology and intellectual property

It is somewhat amusing and appropriate that the cognitive challenges to comprehension and communication of any subtle trinity of "categories" are reflected in the technology through which this is enabled. It is too readily assumed that this can be effectively done through speech or text, however these are enabled by internet communication.

Even the modalities of speech and text reflect somewhat incompatible preferences. Thus the concerns regarding governance articulated in the Gilets Jaunes uprising in France were almost entirely articulated verbally through the media and the unusually extensive commentary there over weeks. The uprising itself was enabled by a high volume of brief communications via social media. There was no implication whatsoever that diagrammatic or geometric articulation -- "mapping" -- would facilitate dialogue and avoid (seemingly endless) repetition.

Perhaps more extraordinary, and especially symptomatic, the well-recognized capacity of the intelligence services to "listen" and record such discourse was in no way matched by any capacity to transform sequential discourse into any more comprehensive and integrative mapping. This inadequacy was compounded by the repeated complaints of authorities that the Gilets Jaunes had failed to articulate the issues with which they were concerned -- suggesting that whilst the intelligence services were capable of "listening", they were totally incapable of "hearing" or "seeing" patterns of significance. Whilst the authorities were assiduous in deploying the "forces d'ordre" to constrain the Gilets Jaunes, they had no ability to deploy any cognitive "forces" to order preoccupations deemed to be such a fundamental challenge by authorities.

Such incapacity is reflected in the preferred modalities of governance in parliamentary assemblies world wide. There is endless scope for speech-making, especially in the antagonistic discourse between right-wing, left-wing and centre parties, assiduously recorded in text. However there is no capacity to transform that text into issue maps to enable dialogue of a higher order. In international assemblies, the irony is all the greater in that, enabled by technology, speech is simultaneously translated between multiple languages, as may be its transcription. There is zero capacity to reflect that simultaneously in cognitive "mapping languages" indicative of evolution of discussion of the theme -- or, more accurately, there is no motivation to use any relevant technology. There is a heavy commitment to repetition and enabling speakers to identify with their indulgence in it.

Efforts to reach agreement, exemplified by the UN Climate Change Conference (Katowice, 2018), focused intensively on reflecting such agreement in linear texts within which every word could be the subject of extensive commentary and dispute. There is no sense in which whatever is implied by "agreement" could be more adequately reflected in a variety of maps -- as is the case with the many boundary disputes. In the case of global issues, this is especially ironic in that the preoccupation is "global" and there is considerably technical familiarity with the advantages and disadvantages of contrasting "projections". Most decision-makers are now familiar with the value of zooming through map representations of different scales in order to "travel around".

A major constraint to comprehension is that all official communication, including that of supportive academic studies and business contracts, is required to conform to the technology of print media -- possibly in terms of length and page size. Images are typically deprecated for reasons of reproduction cost, and most notably because of issues of intellectual copyright. There is almost no scope for animations of any form -- themselves constrained by copyright in all probability. These constraints extend to use of web technology -- with some degree of freedom offered by speech. However (re)use of videos may again be constrained by copyright with which paywalls may be carefully associated.

A further technology constraint is with respect to size. This applies as much in terms of number of pages (or volumes) to make a case, most obviously a legal case, but also in terms of the length of any video (in terms of megabytes). An example of major relevance to global governance is the Trans-Pacific Partnership of over 5,000 pages in length. Comprised of 320 articles and nine annexes, the UN Convention on the Law of the Sea is acknowledged to be one of the longest in history. A striking scientific example is the 15,000 page proof required for the Enormous Theorem (Stephen Ornes, Prize awarded for largest mathematical proof, New Scientist, 9 September 2011). Aside from limited willingness or capacity to engage with such articulations, it may be costly, if not impossible, to ensure their deliverability to all who might choose to explore them (Dreamables, Deniables, Deliverables and Duende: global dynamics "at the table" inspired by dining and wining in practice, 2015).

Further difficulties are evident with respect to systemic complexity and the capacity to engage with it, as argued separately (Comprehension of Numbers Challenging Global Civilization, 2014).

It is appropriate to argue that communication of insights of relevance to coherent global governance is subject to a pattern of constraints of which restrictive intellectual copyright and paywalls are very significant factors. The innovation which is the focus of the Triple Helix model is associated with a process whereby patenting is undertaken competitivelyto inhibit the innovation by others, if not to prevent it. It is fair to argue that there is a particular sense in which knowledge is effectively incarcerated worldwide to a degree that it is necessarily difficult to appreciate (Inhibition of creativity through incarceration of knowledge, 2018). This applies to many of the texts cited here and to images which might have been included.

Engaging experientially with potential: anticipation and nostalgia

This argument referred appreciatively to studies over centuries about how divinity might be comprehended. That argument was reframed in terms of topology and knot-theory (Saiber, 2013). It was stressed that "divinity" could then be understood as a particular comprehension of integrative coherence -- and the quest for it in many contexts, including those of governance and personal individuation. The illusory nature of "divinity" has been assiduously -- and controversially -- clarified from a scientific perspective by Richard Dawkins (The God Delusion, 2006). However that argument can be reinterpreted as indicative of the illusory nature of consensus of any kind (The Consensus Delusion: mysterious attractor undermining global civilization as currently imagined, 2011). The case made by Dawkins can be used as a template for the development of the argument with respect to consensus.

As presented above, rather than the possibility of achieving a permanent "grasp" of coherence, consistent with the drive for possession of intellectual and cultural property, a more appropriate sense of coherence may be associated with its transient manifestation. This can be fruitfully understood as cyclic, namely to be anticipated as recurring -- however fleeting the moment. Whilst things "may come together", this is in a dynamic context in which they will then drift apart. Efforts may indeed be made to "capture" that coherence, and to incarcerate it in some manner -- notably in an agreement. History provides every evidence that this is only temporarily successful and might indeed be better recognized as a form of "delusion" and an indication of mistaken understanding.

The argument can be appropriately made by reference to the experience of astronomical cycles, most notably those celebrated in stone monuments providing a focus on the summer solstice or the winter solstice -- as at Stonehenge and elsewhere. The experience is momentary but it can be anticipated over thousands of years. The contrast to be recognized is with the nature of illusory efforts to express agreement and consensus "in stone". Consensus can indeed be "written in stone", but only when this allows dynamically for its absence, as in such memorial monuments. Frustration with failure in this respect can be usefully explored (Transforming and Interweaving the Ways of Being Stoned: imagination, promise, rocks, memorials, petrification, 2012).

Consensus is then better understood dynamically in terms of "being in phase" momentarily, and of temporary "alignment" -- with the complementary recognition of the systemic function of conditions under which this does not hold. Appreciation of shifting weather conditions offers fruitful metaphors, especially in the light of the questionable preference for "good weather" and on deprecation of "bad weather" (Weather Metaphors as Whether Metaphors: transcending solar illusion via a Galilean-style cognitive revolution? 2015).

Use of virtual reality technology in exploring the relationship between helical forms, as above, offers another illustration of the challenge. Insights from the process cannot be readily communicated since the sense of coherence may be associated as much with interactive engagement with dynamic displays as with anything that can be expressed in the form of screen shot images or selective animations. No singular display can convey what is derived from the capacity to explore the variety within which coherence may emerge as a surprising discovery. Beyond the insights which can be communicated in 2D images, or simple 3D animations, lies the realm of interactive engagement -- a realm to be understood as one of higher dimensionality. It is presumably with such higher dimensionality and its aesthetics that coherence as a strange attractor is more closely associated.

In facilitating premature closure, clearly the role assumed by any "priests of coherence" is a matter of concern in its own right. There would seem to be an unexplored interplay between the confidence engendered by truth, experienced as coherence, and the financial reward resulting from asserting and ensuring exclusive possession of coherence. If the "circle of trust" -- or the ring -- is an "unknot", of what relevance are the insights offered by the strange connectivity of the 3-fold pattern of Borromean Rings?

---

References

David Albertson. Mathematical Theologies: Nicholas of Cusa and the Legacy of Thierry of Chartres. Oxford University Press, 2014 [contents]

P. K. Aravind. Borromean Entanglement of the GHZ State. In: Cohen R.S., Horne M., Stachel J. (eds), Potentiality, Entanglement and Passion-at-a-Distance. Springer, 1997 [abstract]

Samuel Arbesman:

• Why Do Great Ideas Take So Long to Spread? Harvard Business Review, 27 November 2012 [text]
• The Half-life of Facts: why everything we know has an expiration date. Current, 2012

R. Arnkil, A. Järvensivu, P. Koski and Piirainen. Exploring Quadruple Helix: outlining user-oriented innovation models. Final Report on Quadruple Helix Research for the CLIQ project, under the Interreg IVC Programme, 2010.

Ronald H. Atkin:

• From cohomology in physics to q-connectivity in social science. International Journal of Man-Machine Studies, 4, 1972, pp. 139-167. [text]
• Combinatorial Connectivities in Social Systems: an application of simplicial complex structures to the study of large organizations. Birkhauser Verlag, 1977.
• The Methodology of Q-Analysis Applied to Social Systems. Systems Methodology in Social Science Research, 1982 [abstract]
• Multidimensional Man; can man live in 3-dimensional space? Penguin, 1981
• The Mathematical Foundations of Physics. Arima Publishing, 2007 [contents]

Ronald H. Atkin and J. L. Casti. Polyhedral dynamics and the geometry of systems. International Institute for Applied Systems Analysis, 1977

Jacques Attali:

• Noise: The Political Economy of Music. University of Minnesota Press, 1985
• Demain, Qui Gouvernera le Monde? Fayard, 2011

Emma-Louise Aveling, Alex Gillespie and Flora Cornish. A Qualitative Method for Analysing Multivoicedness. Qualitative Research, 15, 2015, 6, pp. 670-687 [text]

Nils A. Baas, Nadrian C. Seeman and Andrew Stacey. Synthesising Topological Links. Journal of Mathematical Chemistry, 53, 2015, 1, pp. 183-199 [text].

A. Bavelas. Some Mathematical Properties of Psychological Space. MIT, 1948.

Stafford Beer:

• The Cybernetic Cytoblast: Management Itself; Progress of Cybernetics. Gordon and Breach, 1969 (Chairman's Address to Congress, International Cybernetics Congress)
• Platform For Change. John Wiley, 1978
• Beyond Dispute: the invention of team syntegrity. John Wiley, 1994

David Bohm:

• Wholeness and the Implicate Order. Routledge, 1980
• On Dialogue. Routledge, 1996

F. P. Brooks. Grasping Reality through Illusion: nteractive graphics serving science. Proceedings of the SIGCHI Conference on Human Factors in Computing Systems, 1988 [text]

Cameron Browne. Taiji Variations: Yin and Yang in multiple dimensions. Computers and Graphics, 31, 2007, 1, pp, 142-146 [abstract]

E. G. Carayannis and D. F. J. Campbell:

• Mode 3 and 'Quadruple Helix': toward a 21st century fractal innovation ecosystem. International Journal of Technology Management, 46, 2009, 3, pp. 201-234
• Triple Helix, Quadruple Helix and Quintuple Helix and how do knowledge, innovation and the environment relate to each other? A proposed framework for a transdisciplinary analysis of sustainable development and social ecology. International Journal of Social Ecology and Sustainable Development, 1, 2010, 1, pp. 41-69
• Mode 3 Knowledge Production 1 in Quadruple Helix Innovation Systems. 2012
• The Quintuple Helix innovation model: global warming as a challenge and driver for innovation. Journal of Innovation and Entrepreneurship, 2012 [text]

E. G. Carayannis and R. Rakhmatullin. The Quadruple/Quintuple Innovation Helixes and Smart Specialisation Strategies for Sustainable and Inclusive Growth in Europe and Beyond. Journal of the Knowledge Economy, 2014 [text].

Simona Cavallini, Rossella Soldi, Julia Friedl and Margherita Volpeet. Using the Quadruple Helix Approach to Accelerate the Transfer of Research and Innovation Results to Regional Growth. Committe of the Regions of the European Union, 2016 [text]

Gregory Chaitin. Meta Math! The Quest for Omega. arXiv, 2004 [text]

Richard Dawkins. The God Delusion. Bantam Books, 2006

Konstantin Y. Degtiarev. Q-Analysis and Human Mental Models: a conceptual framework for complexity estimate of simplicial complex in psychological space. 2011 [text]

Riane Eisler. The Chalice and The Blade: our history, our future. Harpercollins, 1987 [summary]

Erik B. Erhardt. The Borromean Rings. Franklin Pierce College, 1997 [text]

Myfanwy Ella Evans. Three-Dimensional Entanglement: knots, knits and nets. Australian National University, January 2011 [text]

Nick Green. Axioms from Interaction of Actors Theory. Kybernetes: the international journal of systems and cybernetics, 33, 2004, 9/10 [text]

D. Hilbert and S. Cohn-Vossen. Geometry and the Iimagination . Chelsea Publishing Group, 1952.

Hugh Nelson Howards. Forming the Borromean Rings out of Arbitrary Polygonal Unknots. arXiv.org, 12 June 2014 [text]

Louis H. Kauffman:

• Laws of Form: an exploration in mathematics and foundations [rough draft]
• The Borromean Rings: a tripartite topological relationship. 2006 [text]
• Knot Logic and Topological Quantum Computing with Majorana Fermions. arXiv, 20 January 2013 [text]

Jacky Legrand. How far can Q-analysis go into social systems understanding? [text]

Hans Lenk. Grasping Reality: an interpretation-realistic epistemology. World Scientiic, 2003 [contents]

Marshall McLuhan. The Medium is the Message. Bantam Books, 1967

Alexander D. Mednykh. The Three-Dimensional Universe, Where We Are Not Living. Science, 8, 30 May 2006 [text]

Gordon Pask and Gerard de Zeeuw. Interactions of Actors: theory and some applications. 1992 [text]

Steven M. Rosen:

• Dreams, Death, Rebirth: a multimedia topological odyssey into alchemy's hidden dimensions. Chiron, 2014 [summary]
• The Self-evolving Cosmos: a phenomenological approach to nature's unity-in-diversity. World Scientific, 2008 [summary]
• Topologies of the Flesh. Ohio University Press, 2006 [summary]
• Dimensions of Apeiron: a topological phenomenology of space, time and individuation. Value Inquiry Book Series of Editions Rodopi, 2004 [summary]
• The Moebius Seed: a visionary novel of planetary transformatio. Stillpoint, 1985 [summary]

Arielle Saiber. Canto XXXIII: The Song of the Return. In: Allen Mandelbaum (Ed.), Lectura Dante Paradiso, University of California Press, 2019

Arielle Saiber and Aba Mbirika. The Three Giri of Paradiso XXXIII. Dante Studies, 131, 2013, pp. 237-272 [text]

Michael Schiltz:

• Space is the Place: the Laws of Form and social systems. Thesis Eleven, 88, 2007, 1 [abstract]
• Form and Medium: a mathematical reconstruction. Image and Narrative, [text]

E. A. Shanahan, M. K. McBeth and M. D. Jones. Denouement?: The Science of Stories. Palgrave Macmillan, 2014 [abstract]

George Spencer-Brown. Laws of Form. Allen and Unwin, 1969 [summary]

C. Mao, W. Sun and Nadrian C. Seeman. Assembly of Borromean rings from DNA. Nature, 386, 1997, 6621, pp. 137-138 [citations]

Raymond Talli. Of Time ad Lamentation: reflections on transience. Agenda, 2017

Tom Verhoeff and Tom Verhoeff. Three Families of Mitered Borromean Ring Sculptures. Proceedings of Bridges 2015: Mathematics, Music, Art, Architecture, Culture [text]

Alexander Wendt. Quantum Mind and Social Science: unifying physical and social ontology. Cambridge University Press, 2015

M. V. Zhukov, et al. Bound State Properties of Borromean Halo Nuclei. Elsevier, 1993

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License. For further updates on this site, subscribe here